Заглавная страница Избранные статьи Случайная статья Познавательные статьи Новые добавления Обратная связь FAQ Написать работу КАТЕГОРИИ: ТОП 10 на сайте Приготовление дезинфицирующих растворов различной концентрацииТехника нижней прямой подачи мяча. Франко-прусская война (причины и последствия) Организация работы процедурного кабинета Смысловое и механическое запоминание, их место и роль в усвоении знаний Коммуникативные барьеры и пути их преодоления Обработка изделий медицинского назначения многократного применения Образцы текста публицистического стиля Четыре типа изменения баланса Задачи с ответами для Всероссийской олимпиады по праву 
Мы поможем в написании ваших работ! ЗНАЕТЕ ЛИ ВЫ?
Влияние общества на человека
Приготовление дезинфицирующих растворов различной концентрации Практические работы по географии для 6 класса Организация работы процедурного кабинета Изменения в неживой природе осенью Уборка процедурного кабинета Сольфеджио. Все правила по сольфеджио Балочные системы. Определение реакций опор и моментов защемления |
Laboratory work № 2. Measuring of Yung modulus of metalsСодержание книги
Поиск на нашем сайте
The aim: to test Hook Law; to determine Modulus of Yung of steel wire. Instrumentation and appliances: a slide gauge, a ruler, an indicator of lengthening, a set of weights, wire.
Introduction
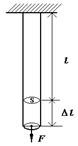
Any body deforms under external stresses, i.e. changes in size and shape. When the action of force stops and the body restores its shape and size the deformation is called elastic. Under plastic deformation shape and size of a body is not restored when external stresses is eliminated. Elastic deformation occurs when external force that creates this deformation doesn’t extend a certain limit which is called elastic limit. Under the effect of applied loading only insignificant change in the distances between atoms ore crystal clusters turning occurs. When stretching the distance between the crystal atoms grows and this distance decreases when compressing. For this case the balance of attraction and repulsion forces is violated, that is why the displaced atoms in the result of the action of attraction or repulsion forcers return to the initial state of equilibrium, and the crystals regain their original size and shape. Let us apply external force F to the rigid rod fixed at one end (fig. 6.1). Let the initial length l increased by Δ l. The ratio of the force to the cross section area - S is called the mechanical stress:
For a cylinder specimen (wire) of the diameter
and the mechanical stress is:
The value:
is called relative deformation that is the ratio of absolute lengthening
Figure 6.1
The experiment proves that linear dependency occurs between σ and ε in elastic deformation area. In fig.6.2 OA region is the elastic deformation area where Hook’s Law is true:
In the AB region a plastic deformation takes place, i.e. in the case when
Figure 6.2
Relation (6.5) proves Hook’s Law, where E is elasticity module or Yung module that characterizes the elastic properties of a material. Yung module is equal to mechanical stress at which the rod length is doubled. The value of Yung’s modulus basically is determined by the type of crystal lattice, i.e. by the forces of atomic bonds. The value of Yung’s modulus for some materials is shown in the table 6.1.
Table 6.1
Experimental device
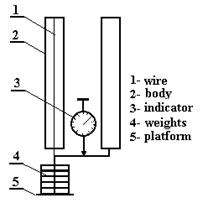
The experimental device is shown in fig.6.3.
Figure 6.3
Wire 1 is fixed on body 2. Platform 5 is fixed to the end of the wire. Weights 4 are placed on the platform. Lengthening of the wire is measured with the indicator of lengthening 3.
Experimental part
1. Measure the diameter of the wire with the slide gauge and the length of the wire with a ruler. 2. Load the wire with 2÷3 weights to make it straight. 3. Fix the zero rotating the indicator scale. 4. Measure the lengthening of the wire Δ l gradually loading it with the weights. Force F applied to the wire equals the sum of weights being loaded to the wire. Put down the results of the measurements m, F, Δ l in a table 6.2. The length of the wire and its diameter are l = 1100 mm, d = 0,6 mm. Calculate mechanical stress and relative deformation according to formula 6.3 and 6.4. Put down the results in table 6.2.
Table 6.2
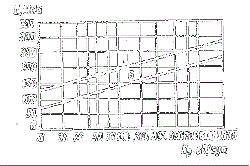
5. Plot the dependency of σ = f(ε) accordingly to fig. 6.4, i.e. draw the best straight line lying in the maximum density of experimental points. Choose any of two points 1 and 2 (but not from the table) to which values of
Figure 6.4
6. Calculate modulus of Yung by the formula:
Having such a method of determining the coefficient of proportionality (fig.6.4) between any values that laniary are related one to another, the all the totality of experimental data but not accidental value of any measurement is used. Using the data from table 6.1 determine the material of the wire. 7. Using the theory of errors estimate relative error of measurement Modulus of Yung by the formula:
where the value δl calculate according to the formula:
where δ = 0.01 mm;
6. On the base of relation σ = f(ε) make the conclusion if the Hook Law is true. Control questions
1. What is the determination of eelastic and plastic deformations? 2. What is the determination of the mechanical stress and the relative deformation? 3. What type is the graph of experimental dependence 4. What is the sens of Hook Law? 5. What is the physical sans of Yung modulus?
Literature
1. И.В. Савельев Курс общей физики. М.- Наука. 1982. 2. Ю.М. Лахтин, В.П. Леонтьева Материаловедение. М.- Машиностроение. 1972. 3. Н.И. Кошкин, М.Г. Ширкевич Справочник по элементарной физике. М.- Наука.1990.
Authors: M.I. Pravda, the reader, candidate of physical and mathematical sciences. Reviewer: S.P. Lushchin, the reader, candidate of physical and mathematical sciences. Approved by the chair of physics. Protocol № 3 from 01.12.2008.
|
||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
|
Последнее изменение этой страницы: 2016-04-08; просмотров: 555; Нарушение авторского права страницы; Мы поможем в написании вашей работы! infopedia.su Все материалы представленные на сайте исключительно с целью ознакомления читателями и не преследуют коммерческих целей или нарушение авторских прав. Обратная связь - 3.145.107.223 (0.009 с.) |

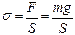 . (6.1)
. (6.1)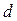 the cross section aria is:
the cross section aria is: (6.2)
(6.2)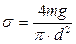 . (6.3)
. (6.3)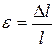 (6.4)
(6.4) to initial length
to initial length  .
.
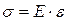 (6.5)
(6.5) residual deformations εr originates as soon as external forces stop acting upon. Stress
residual deformations εr originates as soon as external forces stop acting upon. Stress  is called limit of elasticity of the material.
is called limit of elasticity of the material.
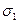 ,
, 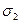 and
and 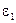 ,
, 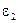 accordingly.
accordingly.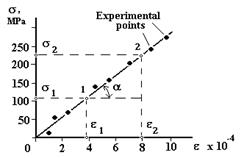
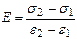 (6.6)
(6.6) , (6.7)
, (6.7)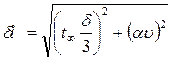 , (6.8)
, (6.8)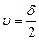 . According to the formula:
. According to the formula: (6.9) calculate the values: Dl (
(6.9) calculate the values: Dl (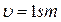 ), Dm (
), Dm ( ), Dg (
), Dg ( ), Dp (
), Dp ( ), Dd (
), Dd (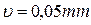 ).
).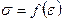 ?
?