Заглавная страница Избранные статьи Случайная статья Познавательные статьи Новые добавления Обратная связь FAQ Написать работу КАТЕГОРИИ: ТОП 10 на сайте Приготовление дезинфицирующих растворов различной концентрацииТехника нижней прямой подачи мяча. Франко-прусская война (причины и последствия) Организация работы процедурного кабинета Смысловое и механическое запоминание, их место и роль в усвоении знаний Коммуникативные барьеры и пути их преодоления Обработка изделий медицинского назначения многократного применения Образцы текста публицистического стиля Четыре типа изменения баланса Задачи с ответами для Всероссийской олимпиады по праву 
Мы поможем в написании ваших работ! ЗНАЕТЕ ЛИ ВЫ?
Влияние общества на человека
Приготовление дезинфицирующих растворов различной концентрации Практические работы по географии для 6 класса Организация работы процедурного кабинета Изменения в неживой природе осенью Уборка процедурного кабинета Сольфеджио. Все правила по сольфеджио Балочные системы. Определение реакций опор и моментов защемления |
Principal concepts of the theory of errorsСодержание книги
Поиск на нашем сайте
We can't define the true values of a physical quantity. We can define only the interval (x min, x max) of the investigated quantity with some probability a. For example: we can affirm, that students' height may be defined between 1.5 m and 2.0 m with probability of 0.9. Then we can prove, that students' height may be defined between 1.6 m and 1.8 m with smaller probability of 0.6 and so on. Value of this interval is called the entrusting interval. On fig.2.1 interval of quantity being investigated x is represented.
Figure 2.1
Where x is the most probable value of quantity being measured; Dx is the half width of the entrusting interval of the measured quantity with probability of a. Therefore we can estimate, that true value of the measured quantity may be defined as x = x or If a quantity x has been measured n times and x1, x2,..., xn are the results of the individual measurements then the most probable measured value or the arithmetic mean is:
The deviation
is called the mean accidental deviation of the measurements. Mean root square is defined as
where t – Student’s constant for definite a and n. The ratio of
is called the relative error of measurement and is usually expressed in percents:
Errors of instruments
Absolute error of instrumental d is a deviation
where a is an index of an instrument; X is the true value of the quantity measured. Typically d is quantity of the instruments minimum value scale. For example: the ruler error is d = 1 mm. Relative error of the measurement is the ratio of
It is usually expressed in percent
Brought error of the measurement or precision class is the ratio
expressed in percent. D is maximum value on the instrument scale. For example: electric current is measured by the instrument with interval 0 ÷ 1 A, precision class is 0.5. This means, that D = 1 A, g = 0.5 %, and
If the instrument shows 0.3 A, then
Error of table quantities, count and rules of approximations
1. The error of table quantity is defined as
where, α is probability; v is half price of category from last significance figure in table quantity. For example: quantity p may be 3.14. In this case v = 0.005 and
If quantity p is 3.141 and v = 0.0005 then
and so on. 2. Error of count may occur when we measure quantity by an instrument. Typically, the error of count is half price of minimum value of instruments scale. For example a ruler has error of count vl=0.5 mm. 3. Rules of approximation: quantity x may be approximated only to two significant figures, if the first significant figure is 1 or 2. In other cases, the quantity is approximated only to one significant figure. For example: x = 0.01865, approximated quantity is 0.019; x = 0.896, approximated quantity is 0.9 and so on.
Errors of direct measurement
Errors of direct measurements are defined as
if there is one measurement (n = 1). And
if there are several measurements (n > 1). In these equations t¥ is Student's constant, it may be defined from the table on the crossing of line with n and column with a; d is error of an instrument; v is error of count, v =d/2. For example: the length of a body was measured three times:
The error of this measurement will be:
The relative error is
The final result is x = (13.3 + 0.5) mm, a= 0.7, E = 3.6 %.
Errors of indirect measurements
Let y be indirectly measured quantity, it is defined as y = f(x1,x2,..., xn). x1,x2,..., xn is defined as the direct measurements. 1. Errors of indirect measurements are defined as
if the functional dependence of investigated quantity is a polynomial. 2. Errors of indirect measurements are defined as
if the functional dependence of investigated quantity, is a monomial and we can define Dy as: 1. If the functional dependence is
Then
2. If functional dependence is
Then
and
The final result:
Graph presentation of the experimental results
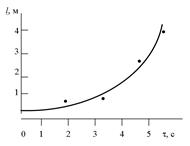
Graph is built on the millimeter paper. In fig.2.2 you can see an example of graph.
Figure 2.2
The experimental curve is drawn through the experimental points. This curve describes the experimental data.
Control questions
1. Definition of direct and indirect measurements. Examples. 2. Definition of the most probable value of the measured quantity x. 3. What is called a relative error? 4. What is called an accidental deviation? 5. What is the equation of square mean of errors? 6. How do we define errors of instruments? 7. How do we define errors of table quantities and count errors? 8. Rules of approximation. 9. What is the equation of errors of direct measurements? 10. What is the equation of errors of indirect measurements?
Authors: S.P. Lushchin, the reader, candidate of physical and mathematical sciences. Reviewer: S.V. Loskutov, professor, doctor of physical and mathematical sciences. Approved by the chair of physics. Protocol № 3 from 01.12.2008.
|
|||||||||||||||||
|
Последнее изменение этой страницы: 2016-04-08; просмотров: 453; Нарушение авторского права страницы; Мы поможем в написании вашей работы! infopedia.su Все материалы представленные на сайте исключительно с целью ознакомления читателями и не преследуют коммерческих целей или нарушение авторских прав. Обратная связь - 18.216.92.5 (0.006 с.) |


 D x, with probability a,
D x, with probability a, .
. (2.1)
(2.1) is called the accidental error (deviation) of a single measurement.
is called the accidental error (deviation) of a single measurement. (2.2)
(2.2) (2.3)
(2.3)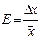 (2.4)
(2.4) . (2.5)
. (2.5) , (2.6)
, (2.6) . (2.7)
. (2.7) . (2.8)
. (2.8) , (2.9)
, (2.9) .
. .
. , (2.10)
, (2.10) .
.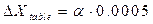
 , (2.11)
, (2.11)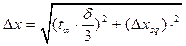 , (2.12)
, (2.12)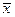 =13.266, n=3, t=1.4, t¥=1, d=1 mm.
=13.266, n=3, t=1.4, t¥=1, d=1 mm.

 .
. (2.13)
(2.13) (2.14)
(2.14) . For example:
. For example: then
then
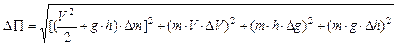
 ,
,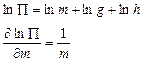

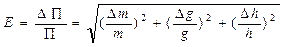

 .
.