Заглавная страница Избранные статьи Случайная статья Познавательные статьи Новые добавления Обратная связь FAQ Написать работу КАТЕГОРИИ: ТОП 10 на сайте Приготовление дезинфицирующих растворов различной концентрацииТехника нижней прямой подачи мяча. Франко-прусская война (причины и последствия) Организация работы процедурного кабинета Смысловое и механическое запоминание, их место и роль в усвоении знаний Коммуникативные барьеры и пути их преодоления Обработка изделий медицинского назначения многократного применения Образцы текста публицистического стиля Четыре типа изменения баланса Задачи с ответами для Всероссийской олимпиады по праву 
Мы поможем в написании ваших работ! ЗНАЕТЕ ЛИ ВЫ?
Влияние общества на человека
Приготовление дезинфицирующих растворов различной концентрации Практические работы по географии для 6 класса Организация работы процедурного кабинета Изменения в неживой природе осенью Уборка процедурного кабинета Сольфеджио. Все правила по сольфеджио Балочные системы. Определение реакций опор и моментов защемления |
Questions and tasks for selfcontrol.Содержание книги
Поиск на нашем сайте
1. Give the definition for “ion product of water”. What correlation exists between this value and constant of the water. 2. How is the numeral value of water ion product determined. Does it depend on temperature? 3. How can you determine the ion concentration of OH- (H+) if the concentration of H+ (OH-) ions is known? 4. Is the given statement right: “In the solution of strong acid (alkali) OH- (H+) ions are contained”? Prove the answer. 5. What relationship exists between concentration of H+ (OH-) ions in the solutions of strong and weak acids and bases and their molar concentration? 6. Give the definition for hydrogen ion exponent (pH) and hydroxide ion exponent (pOH). What is their sum equal to? 7. What values do the concentrations of ions H+ (OH-) and pH (pOH) in different mediums accept? 8. Which electrolytes solutions will have the lowest value of pH if their molarities are the same: a) HCl or CH3COOH b) HNO2 or HClO c) HCl or KOH. 9. Give the definition for hydrolysis. Describe this process as: a) covalent and ionic; b) by cation and by anion c) simple and multistage d)reversible and unreversible. Give the examples. 10. How does the addition of hydrolyzed salt, such as ZnCl2, K2CO3, Al2S3 influence on the equilibrium: H2O ↔ H+ + OH- and pH value 11. Choose the salt whose pH of solution will be more: a) NaNO3 or NaNO2 b)CH3COOK or CH3COONH4 c) KClO or KClO3. 12. Is it possible with the help of acid-base indicator to distinguish the salts solution: a)NaClO4 and NaClO b) KI and NH4I c) KClO3 and KCl d) Na2CO3 and Zn(NO3)2, i) BeCl2 and BaCl2 F) MgSO4 and K2SO4. 13. Give the definition of “ hydrolytic equilibrium”. 14. Give the definition for hydrolysis degree and hydrolysis constant. Do them depend on salt nature, solution concentration, temperature? 15. What formula expresses the relationship between constant and degree of hydrolysis. 16. What relationship exists between hydrolysis constant and dissociation constant of weak electrolyte? 17. What salt is hydrolyzed in a greater extent: a) KCN or KSCN (K(HCN) = 7,9∙ 10-10, K(HSCN) = 1,4∙10-1), b) Na2S or NaHS (K1a = 6∙10-8, K2a = 1∙10-14). Prove the answer with calculations. 18. How can you explain the interincrease of hydrolysis of two salts when their solutions are mixed? In what cases this phenomenon is contemplated. 19. Solve for pH of 0,05% HNO3 solution if ρ(s-n) = 1 g/ml; α(HNO3) = 100%. The answer: 2,1 20. Solve for molarity of CH3COOH solution whose pH is equal to 3. Ka = 1,75 · 10-5. The answer: 0,06 mol/L. 21. Solve for pH of solution, obtained by dilution with water of 0,01L of 30% NaOH solution(ρ = 1,328 g/ml) till 0,75L. α = 70%. The answer: 13,0 22. Solve for pH of solution in 3 L of which 8,1·10-4 Mol of OH- ions are contained. The answer: 9,2. 23. Solve for pH of solution in 0,4 L of which 0,39 Mol of NH3 are contained, if Kb = 1,77·10-5. The answer: 11,6. 24. Solve for pH of HCN solution if a) α = 0,0265%, b) 0,0084%. The answer: 5,53; 5,03. 25. Solve for [H+] and pOH of 0,2 N solution of weak one-basic acid whose dissociation degree is equal to 0,03.The answer: [H+] = 6,0·10-3 mol/l;pOH = 11,78. 26. Solve for pH of solution obtained by mixing of 25 ml of 0,5 M HCl solution and 10 ml of 0,5 M NaOH solution and 15 ml of H2O. The answer: 0,82. 27. Solve for pH of CH3COOH solution in which it’s mass fraction is 0,6%. What volume of water must we add to this solution with volume of 1 L to obtain pH value equal to 3. The answer: 2,87; 0,8 L 28. Solve for hydrolysis constant of NH4Cl if Kb = 1,77 · 10-5. The answer:5,65·10-10. 29. Solve for hydrolysis constant of following salts: HCOONa, HCOONH4, NH4NO3 if Ka = 1,77·10-4, Kb = 1,77·10-5. The answer:5,64·10-11, 3,20·10-6; 5,65·10-10. 30. Solve for hydrolysis degree and pH of 0,005 N KCN solution if Ka = 4,9·10-10. The answer: 0,063; 10,5.
Sample of test card on the topic “Autoprotolysis of water.Hydrogen and Hydroxyl ion exponents. Salt hydrolysis” Point to pH of 0,01 N HCl solution if α = 80%. a) 2,2 b) 11,9 c) 10-2 d) 8·10-3
2. Point to pH of 0,001 M CH3COOH solution if Ka = 1,8 ·10-5 a) 3,87 b) 11,13 c) 1,8 · 10-3 d) 1,35·10-4
3. Calculate Khyd. of KF, if Ka = 6,5 ·10-4 a) 1,54·10-12 b) 1,54·10-11 c) 1,54·10-10 d) 1·10-14
4 pH value and character of the medium in aqueous solution of K2CO3 are the following: a) equal to 7, neutral c) more than 7, alkaline b) less than 7, acidic d) less than 7, alkaline
5. Choose the formula for [H+] calculation: 10-14 α a) —— b) (OH-) c) C∙ α2 d) —— [OH-] Ka
The answers to tasks of card sample Number of question 1 2 3 4 5 Code of answer a a b c a
Class 4 Topic: Buffer solutions. Heterogeneous equilibrium Buffer solutions The solutions of weak acids or weak bases with their salts possess the buffer properties: buffer solutions can be diluted without changing of H+ concentration (pH), buffer solution also tends to keep pH constant even when small amounts of strong acid or base are added to them. The expression for the concentration of H+ and pH in buffer solutions of weak acid HAn and its salt with strong base is applied: C(HAn) [H+] = Ka —————; C(An-) C(An-) C(Salt) pH = pKa + lg ——— = pKa + lg ————, C(HAn) C(acid) where pKa = -lgKa.
In the same way we can calculate the concentration of OH- and pH in buffer solutions of weak bases (KtOH) and their salts with strong acid: C(KtOH) [OH-] = Kb —————; C(Kt+)
C(Kt+) C(Salt) pH = 14 – pKb - lg ——— = 14 – pKb -lg ————, C(KtOH) C(base)
If the buffer solution consists of medium salt (Kt2An) and acidic salt (KtHAn) of bi-basic acid H2An, pH is calculated as: C(An2-) pH = pKa2 + lg ———, where pK2 = - lgK2, exponent of Kafor C(HAn-) H2An for the second step.
If the buffer mixture is formed by acidic salts (Kt2HAn and KtH2An) of three-basic acid H3An, the following equation is used:
C(HAn2-) pH = pK2 + lg ————, C(H2An-) Every buffer solution have its own particular capacity. Number of equivalents of acid or base necessary for changing of pH of 1 L of buffer solution by one unit, is called buffer capacity (B): ν(x) B(x) = ————, when V(buf. sol.) = 1L, or the net equation pH1 - pH2 ν(x) B(x) = —————————, where X –acid or base. V(buf. sol.) ∙│ΔpH│
Tasks with their solutions.
1. 15 ml of 0,03 M-solution HCOOH are added to 15 ml of 0,03 M-solution HCOONa. Calculate pH of derived buffer solution. Ka = 1,77∙10-4.
V1 = V2 = 15 ml The first way:
C1 = C2 = 0,03 m/L [H+] = Ka.C(HCOOH) / C(HCOO-) pH =-lg[H+] Ka = 1, 77∙10-4. 1) [HCOO-] in buf.sol.= 15 ∙ 0,003/30 = 0,015M/L
pH -? 3) [H+] = 1,77∙10-4 ∙ 0,015/0,015 = 1,77∙10-4 4) pH = -lg1, 77∙10-4 = 4 - lg 1,77 = 3,75 The second way: It can be simplified as: [HCOO-] 15∙0,03 pH = pKa + lg————— = 3,75 + lg——— =3,75 [HCOOH] 15∙0,03 The answer: 3,75 2. How will pH of buffer mixture formed by 0,1M solution of CH3COOH and by 0,1 M solution of CH3COONa change if we add: a) 0,001M solution of HCl b) 0,001M NaOH solution? Ka = 1,8∙10-4. Solution: In buffer mixter C(acid) 0,1 [H+] = Ka ———— = 1,8 ∙10-5∙—— = 1,8 ∙10-5 С(salt) 0,1 pH = pKa = - lg1,8∙10-5 = 4,75 If the HCl acid is added: 0,001 0,001 0,001 CH3COONa + HCl = CH3COOH + NaCl
[CH3COOH] = 10-1 + 10-3 = 1,01∙10-1 (Mol/L) [CH3COONa] = 10-1 - 10-3 = 0,99∙10-1 (Mol/L) [H+] =1,8∙10-5∙1,02 = 1,84 ∙10-5(Mol/L) If the NaOH is added: CH3COOH + NaOH = CH3COONa + H2O [H+] =1,8∙10-5∙0,98 = 1,76 ∙10-5(Mol/L) pH = - lg1,76∙10-5 = 5- 0,24 = 4,76 The answer: pH is practically constant.
3. Calculate the buffer capacity of blood serum by acid, if 10 ml of 0,1 N HCl solution are consumed for titration of 50 ml of serum to change pH from 7,4 to 7,0.
pH1 = 7,4 ν(x) pH2 = 7 B(x) = —————————, V(HClsol) =10 ml V(buf. sol.) ∙│ΔpH│ C(HCl) = 0,1 M/L 1) ν(HCl) = 10∙10-3∙0,1 = 10-3
3) 10-3 B(HCl) = ————— = 0,05 (M/L) 50∙10-3∙0,4 The answer: 0,05 (M/L)
Questions for self-control 1. What mixtures are called the “buffers”? 2. What is called conjugate acid, conjugate base? Give the examples of protolytic reactions. 3. What types are the buffer solution divided into? Give the examples. 4. Explain, how the buffer solutions work. As the example take the acetic buffer solution. 5. What is the mechanism of the buffer action, take for example ammonia buffer solution. 6. How should we calculate the concentration of H+ -ions in buffer solution of acidic type. 7. How should we calculate the concentration of H+ -ions in buffer solution of basic type. 8. How should you explain the fact: the addition of small amounts of strong acid and bases, and the dilution does not change the acidity of buffer solutions practically. 9. How should you calculate the pH –values in buffer solutions of acidic or basic type. 10. How do you understand: a) buffer capacity of solution b) buffer strength of solution. 11. 1 ml of 0,5M NH3 solution was added to 20 ml of 1% NH4NO3 (ρ = 1 g/ml). Prepared solution was deluted in measured flask till 100 ml. Calculate the pH of obtained solution. Kb=1,8·10-5, pKb=4,75. The answer: 8,56. 12. 15 ml of 0,2 M K2HPO4 solution are added to 25 ml 0,2M KH2PO4 solution. Calculate the pH of obtained solution. Ka1= 7,6·10-3, pKa1= 2,12; Ka2= 6,3·10-8, pKa2 = 7,2; Ka3=1,3·10-12, pKa3=11,87. The answer: 6,98. 13. 15 ml of 0,15M HCOOK solution are added to 12 ml 0,03 M HCOOH solution. Calculate the pH of obtained solution. Ka= 1,8·10-4, pKa= 3,75. The answer: 4,35. 14. Calculate the pH of solution, 500 ml of which contain 1 g of HCOOH and 1 g of HCOOK. Ka= 1,8·10-4, pKa= 3,75. The answer: 3,49. 15. 0,5% solutions of ammonia and ammonium nitrate are mixed in equal amounts. Calculate the pH of the obtained solution whose density is 1 g/ml; Kb= 1,8·10-5, pKb= 4,75. The answer: 9,92. 16. How many ml of 0,2 M Na2CO3 solution can we add to 10 ml of 0,3 M NaHCO3 solution to prepare the solution with pH = 10? Ka1= 4,4·10-7, pKa1= 6,36; Ka2=4,7·10-11, pKa2 =10,33. The answer: 7 ml. 17. How many grams of 0,2 M Na2CO3 should we add to 100 ml of 0,3 M NaHCO3 solution to prepare the solution with pH 10? Ka1= 4,4·10-7, pKa1= 6,36; Ka2= 4,7·10-11, pKa2 =10,33. The answer: 1,49 g.
Heterogeneous equilibrium
In saturated solution of slightly soluble electrolyte KtAn chemical equilibrium is established: KtAn(solid) ↔ Kt+(s-n) + An-(s-n) The equilibrium is quantitatively characterized by the constant of heterogeneous equilibrium, which is called the solubility product (SP). SP(KtAn)=[KT+]∙[An-] = IP(KtAn) ionic product. In saturated solution SP = IP in unsaturated solution SP>IP, in supersaturated solution SP<IP. Concentration of electrolyte X in saturated solution is called solubility (S(x) in mol/l): S(x) = L(x)/M(X), where L(X) – solubility of electrolyte in gram/ l. The relationship between the solubility and the solubility product of electrolyte in dependence on number of ions in a molecule of electrolyte can be presented in the following table:
Tasks with solutions 1. 2,88∙10-6 g of AgI can be dissolved in 1 liter of water. Calculate the SP (AgI).
Data: Solution: V(H2O) = 1 L AgI(s) = Ag+(aq) + I-(aq) m(AgI) = 2,88∙10-6 g SP(AgI) = [Ag+]∙[I-]
SP(AgI) -? [Ag+] = [I-] = C(AgI) = —————— M(AgI)∙V(sol.)
1)V(solution) = V(H2O) = 1 L; M(AgI) = 23 g/M 2) C(AgI) = 1,225∙10-8 Mol/L 3)SP(AgI) = (1,225∙10-8)2 =1,5∙10-16 The answer: 1,5∙10-16
2. Does the precipitate of Fe(OH)3 appear in the solution containing 1,5∙10-16 Mol/L of FeCl3 and 5∙10-5 Mol/L of NaOH? SP = 3,8∙10-38
C(FeCl3) = 1,5∙10-3M/L Fe(OH)3 (s) = Fe3+(aq) + 3OH-(aq) C(NaOH) = 5∙10-5 M/L SP(Fe(OH)3) = [Fe3+]∙[OH-]3 SP(Fe(OH)3) =3,8∙10-38 1) [Fe3+] = C(FeCl3) = 1,5∙10-3M/L
IP><SP -? 3)Fe(OH)3=[Fe3+]∙[OH-]3=1,5∙10-∙(5∙10-5)=1,9∙10-6 4)IP>>SP, consequently, precipitate is formed. The answer: it is formed.
pH =6 Al(OH)3(s) = Al3+(aq) + 3OH-(aq) SP(Al(OH)3 = 5,1∙10-33. 10-14
[Al3+] -? [H+] 1) [H+]= 10-pH= 10-6 (Mol/L) 2) [OH-]=10-14/10-6 = 10-8 (Mol/L) 5,1∙10-33 3) [ Al3+] = ———— =5,1∙10-9 (Mol/L) (10-8)3 The answer: 5,1∙10-9 (Mol/L) Questions for self-control 1. What equilibrium is called heterogeneous? 2. Derive the expression of solubility product (SP) for slightly soluble salt AgCl. 3. Under what conditions does precipitate appear, dissolve, and exist in equilibrium with the solution. Compare IP (ion product) and SP (solubility product). 4. How does the solubility of a substance change in the presence in it’s saturated solution of the common ion? Prove the answer. 5. Derive the formulas for calculating SP if the solubility (S) is known for the cases: a) BaSO4; b) Mn(OH)2 c) Cr(OH)3 d) Mg3(PO4) f) KtnAnm 6. How many grams (g) of CaSO4 are dissolved in 1 litre of water if SP(CaSO4) = 6,1∙10-5? The answer: 1,062g. 7. 0,001215 g of Ca3(PO4)2 are dissolved in 1 litre of water. Calculate SP (Ca3(PO4)2). The answer: 1∙10-25. 8. Compare the solubility of two salts: AgCl and Ag2CrO4. How many times is one salt as soluble as the another? SP(AgCl) = 1,1∙10-10, SP(Ag2CrO4) = 1,6∙10-12. The answer: silver chromate is 7,3 times as soluble as silver chloride in moles, and 16 times in grams. 9. How many grams (g) of BaSO4 are contained in 100 L of saturated solution, if SP(BaSO4) = 1∙10-10. The answer; 0,23 g. 10. How many grams (g) of AgBr are contained in 10 L of saturated solution, if SP(AgBr) = 4∙10-13. The answer: 1,19∙10-3 g. 11. How many grams (g) of NaOH should we add to 20 ml of the solution, containing 5∙10-5 M MgCl2 to precipitate Mg(OH)2? SP(Mg(OH)2) =1,2∙10-11.The answer: 3,9∙10-4 g. 12. How many milliliters of 2M HCl solution should we add to 20 ml of the 3∙10-2 M Pb(NO3)2 solution, to precipitate PbCl2? SP(PbCl2) = 2,4∙10-4. The answer: 0,94 ml. 13. Is CaSO4 precipitated if we add to 0,1 M CaCl2 solution the equal volume of 0,1 M H2SO4 solution. SP(CaSO4) = 6,1∙10-5. The answer: IP = 4,26∙10-4: CaSO4 is precipitated. 14. What is the value of pH when Mg(OH)2 is precipitated from 2∙10-2 M MgSO4 solution? SP(Mg(OH2) = 1,2∙10-11. The answer: 9,38.
|
|||||||||||||||||||||||||||||||
|
Последнее изменение этой страницы: 2020-12-17; просмотров: 121; Нарушение авторского права страницы; Мы поможем в написании вашей работы! infopedia.su Все материалы представленные на сайте исключительно с целью ознакомления читателями и не преследуют коммерческих целей или нарушение авторских прав. Обратная связь - 3.22.242.169 (0.011 с.) |

 Data: Solution:
Data: Solution: 2) [HCOOH] in buf.sol.= 15 ∙ 0,003/30 = 0,015M/L
2) [HCOOH] in buf.sol.= 15 ∙ 0,003/30 = 0,015M/L  Data: Solution:
Data: Solution: B-? 2) ΔpH = pH1 – pH2 = 7,4 –7,0 =0,4
B-? 2) ΔpH = pH1 – pH2 = 7,4 –7,0 =0,4 

 KtAn
(AgCl)
KtAn
(AgCl)



 KtAn2
(PbI2)
KtAn2
(PbI2)



 KtAn3
(Fe(OH)3)
KtAn3
(Fe(OH)3)

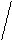 Kt3An2
(Ca3(PO4)2)
Kt3An2
(Ca3(PO4)2)
 5 SP
108
5 SP
108


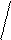 KtnAnm
KtnAnm
 nn∙mm
nn∙mm

 m(AgI)
m(AgI) Data: Solution:
Data: Solution: 2) [OH-] = C(NaOH) = 5∙10-5 M/L
2) [OH-] = C(NaOH) = 5∙10-5 M/L 3. What concentration of Al3+ is necessary to form the precipitate of Al(OH)3 from the solution with pH = 6? SP(Al(OH)3) = 5,1∙10-33.
3. What concentration of Al3+ is necessary to form the precipitate of Al(OH)3 from the solution with pH = 6? SP(Al(OH)3) = 5,1∙10-33. Data: Solution:
Data: Solution: [OH-] = ———; SP(Al(OH)3 )= [ Al3+]∙[OH-]3
[OH-] = ———; SP(Al(OH)3 )= [ Al3+]∙[OH-]3